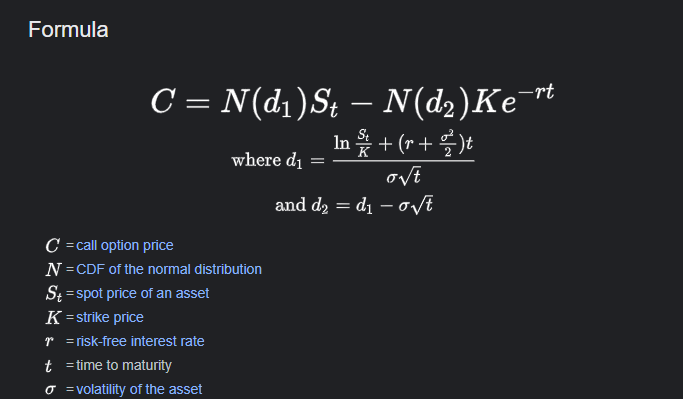
Mastering Profitable Investing: Unleashing the Trillion-Dollar Equation and Options Hedging with Black-Scholes Formula
Introduction
https://chat.openai.com/c/e87467f3-1330-443d-8e68-f225619c9c77
In the fast-paced world of finance, where risks and opportunities coexist, investors constantly seek tools and strategies to navigate the markets successfully. One such powerful tool that has revolutionized the way we perceive and manage financial risks is the Black-Scholes formula. This trillion-dollar equation, developed by economists Fischer Black, Myron Scholes, and Robert Merton, has become a cornerstone for options pricing and hedging strategies in modern investing.
This article delves into the intricacies of the Black-Scholes formula, providing a comprehensive guide on its application and its synergy with options for effective hedging and profitable investing.
Understanding the Trillion-Dollar Equation: Black-Scholes Formula
The Black-Scholes formula is a groundbreaking mathematical model used to calculate the theoretical price of European-style options. Published in 1973, the formula transformed the landscape of financial markets by providing a systematic approach to pricing options, which were previously considered complex and challenging to value.
The key components of the Black-Scholes formula include:
- Stock Price (S): The current market price of the underlying asset.
- Strike Price (K): The pre-determined price at which the option can be exercised.
- Time to Expiry (T): The time remaining until the option’s expiration.
- Risk-Free Interest Rate (r): The theoretical return on a risk-free investment over the option’s time to expiry.
- Volatility (σ): A measure of the underlying asset’s price fluctuations.
The formula itself is expressed as:
C=S0N(d1)−Ke−rTN(d2)
Where:
- C is the Call option price.
- S is the current stock price.
- (1)N(d1) and (2)N(d2) are cumulative distribution functions of the standard normal distribution.
- K is the strike price.
- r is the risk-free interest rate.
- T is the time to expiration.
- σ is the volatility.
For a Put option, the formula is adjusted accordingly:
P = Ke−rTN(−d2)−S0N(−d1)
It’s essential to note that the Black-Scholes formula assumes a constant risk-free interest rate, no dividends, and that market movements follow a log-normal distribution. While these assumptions have limitations, the formula provides a valuable starting point for options pricing.
Using the Black-Scholes Formula for Profitable Investing
- Options Pricing and Valuation:
- The Black-Scholes formula is instrumental in determining the fair market value of options, guiding investors in making informed decisions regarding buying or selling options.
- By plugging in the relevant parameters, investors can calculate the theoretical price of options and compare it with the market price. Discrepancies between the two may present trading opportunities.
- Implied Volatility Insights:
- The formula allows investors to reverse engineer and calculate implied volatility. This is the market’s expectation of future volatility embedded in the option’s price.
- Comparing implied volatility with historical volatility can reveal potential mispricings. An investor may identify options with implied volatility significantly higher or lower than historical volatility, suggesting potential trading opportunities.
- Risk Management:
- Investors can use the Black-Scholes model to assess the impact of changes in different parameters on option prices. Sensitivity analysis helps in understanding how the option’s value responds to variations in factors such as volatility, time to expiry, and interest rates.
- This insight aids in constructing a well-balanced and diversified portfolio, aligning with an investor’s risk tolerance and financial objectives.
Options Hedging Strategies with Black-Scholes
Hedging is a risk management strategy that involves using financial instruments to offset potential losses in an existing investment. The Black-Scholes formula plays a pivotal role in devising effective options hedging strategies. Here are some popular hedging techniques:
- Delta Hedging:
- Delta measures the sensitivity of an option’s price to changes in the underlying asset’s price. Delta hedging involves establishing a position in the underlying asset to offset the option’s delta and minimize exposure to directional market movements.
- For a call option, a delta of 0.70 implies that for every $1 increase in the underlying stock price, the call option’s price will increase by $0.70. To hedge, an investor could short the equivalent delta in the stock.
- Gamma Hedging:
- Gamma measures the rate of change of an option’s delta concerning changes in the underlying asset’s price. Gamma hedging involves adjusting the delta hedge as the underlying asset’s price fluctuates.
- Investors dynamically rebalance their hedge positions, buying or selling more of the underlying asset to maintain a neutral delta position. This strategy is particularly useful for managing risks in highly volatile markets.
- Vega Hedging:
- Vega measures an option’s sensitivity to changes in implied volatility. Vega hedging involves adjusting the position in the underlying asset or options to account for changes in implied volatility.
- If implied volatility increases, the investor might increase their position in the underlying asset or options to compensate for potential losses in the option’s value due to higher volatility.
- Theta Hedging:
- Theta measures an option’s sensitivity to time decay. Theta hedging involves adjusting the position to account for the diminishing time value of the option as it approaches expiration.
- Investors might reduce their exposure to time decay by adjusting the delta hedge, either by reducing the size of the position or by shifting to options with longer expiration dates.
Real-World Application of Black-Scholes and Options Hedging
To illustrate the practical application of the Black-Scholes formula and options hedging, consider the following scenario:
Scenario: An investor holds a portfolio of stocks and is concerned about potential market downturns. To protect against losses, the investor decides to use options and the Black-Scholes formula for hedging.
- Portfolio Composition:
- The investor holds a diversified portfolio of tech stocks with a current value of $1 million.
- Concerned about potential downside risk, the investor decides to hedge the portfolio using put options.
- Options Selection:
- The investor selects out-of-the-money put options on an index that closely mirrors the tech sector.
- The investor chooses options with a maturity of three months, as this aligns with the investor’s short to medium-term risk outlook.
- Black-Scholes Calculation:
- The investor uses the Black-Scholes formula to calculate the theoretical price of the selected put options.
- Parameters include the current stock index level, the strike price of the put options, the risk-free interest rate, time to expiry, and implied volatility.
- Delta Hedging:
- The investor calculates the delta of the put options to determine the required hedge position in the underlying index.
- The investor dynamically adjusts the hedge position as the market moves, maintaining a neutral delta to mitigate directional risk.
- Monitoring and Adjusting:
- Regularly monitoring the options portfolio and underlying assets allows the investor to make necessary adjustments.
- If implied volatility
increases or decreases, the investor can adjust the hedge position accordingly to account for changes in the options’ values due to volatility shifts.
- Gamma and Vega Hedging:
- The investor incorporates gamma and vega hedging into the strategy to address changes in the underlying asset’s price and implied volatility.
- As the market evolves, the investor adjusts the hedge dynamically, ensuring that the portfolio remains resilient to market fluctuations.
- Theta Hedging and Roll-Down Strategy:
- Theta decay becomes a crucial consideration as the options approach expiration. The investor may implement a roll-down strategy, rolling the options position to a new maturity date to capture additional time premium.
- By continuously managing theta decay, the investor optimizes the cost-effectiveness of the hedging strategy.
- Profitable Outcomes:
- In the event of a market downturn, the protective put options provide a hedge, offsetting losses in the stock portfolio.
- If the market remains stable or experiences a modest uptrend, the cost of the protective puts is offset by gains in the underlying stocks, allowing the investor to participate in the market’s upside.
- Risks and Considerations:
- While options hedging strategies can mitigate risks, they also come with costs, including the premiums paid for options.
- Investors need to carefully consider transaction costs, bid-ask spreads, and the impact of market frictions on the overall profitability of the strategy.
- Continuous Evaluation and Adjustments:
- Successful options hedging requires ongoing monitoring and adjustments. Market conditions, economic factors, and geopolitical events can impact the effectiveness of the hedging strategy.
- Regularly reassessing the portfolio, updating assumptions, and adapting to changing market dynamics are essential for optimizing the risk-return profile.
Integration of Black-Scholes and Options Hedging in Investment Portfolios
- Diversification and Risk Management:
- The Black-Scholes formula and options hedging strategies provide investors with powerful tools for diversifying and managing risks in their portfolios.
- By incorporating options with different maturities and strike prices, investors can tailor their hedging strategies to specific risk profiles and market outlooks.
- Portfolio Tailoring for Market Conditions:
- In bullish markets, investors may opt for covered call strategies to generate income while participating in potential upside.
- During periods of heightened uncertainty, protective put options can act as a safety net, limiting downside risk and preserving capital.
- Adaptability to Market Sentiment:
- Options provide investors with the flexibility to adjust their exposure based on changing market sentiment.
- Bullish, bearish, and neutral strategies can be implemented, allowing investors to navigate various market conditions and profit from different scenarios.
- Enhanced Risk-Adjusted Returns:
- By judiciously integrating options strategies within a broader investment portfolio, investors can enhance risk-adjusted returns.
- The ability to manage risk dynamically allows for a more efficient allocation of capital, maximizing returns for a given level of risk.
- Educational Imperative:
- Successful implementation of options strategies requires a deep understanding of the Black-Scholes formula, options pricing, and various hedging techniques.
- Investors should continuously educate themselves on market dynamics, implied volatility, and evolving financial instruments to make informed decisions.
- Technological Tools and Platforms:
- Utilizing advanced technological tools and trading platforms can streamline the implementation of options strategies.
- Algorithmic trading, options analytics software, and real-time market data contribute to efficient decision-making and execution.
- Professional Advice:
- Given the complexity of options strategies and the financial markets, seeking advice from financial professionals, including financial advisors and options specialists, can be invaluable.
- Professional guidance can help tailor strategies to individual financial goals, risk tolerances, and time horizons.
Conclusion
The trillion-dollar equation, the Black-Scholes formula, and options hedging strategies offer investors a sophisticated framework for navigating the complexities of financial markets. By understanding the principles behind options pricing and applying effective hedging techniques, investors can manage risk, enhance returns, and adapt to various market conditions.
Successful implementation requires a commitment to continuous learning, a keen awareness of market dynamics, and the ability to adapt strategies based on evolving economic factors. As with any investment strategy, there are risks involved, and careful consideration, thorough analysis, and prudent decision-making are essential components of a successful options and hedging approach.
In conclusion, the synergy between the Black-Scholes formula and options hedging provides investors with a powerful toolkit to not only protect their portfolios but also capitalize on market opportunities. With a solid understanding of these concepts and a commitment to ongoing education, investors can harness the potential of options and the Black-Scholes formula for profitable and resilient investing in the ever-changing landscape of financial markets.
Feb 27, 2024 #2 on TrendingThe most famous equation in finance, the Black-Scholes/Merton equation, came from physics. It launched an industry worth trillions of dollars and led to the world’s best investments. Go to https://www.eightsleep.com/veritasium and use the code Veritasium for $200 off your Pod Cover. If you’re looking for a molecular modeling kit, try Snatoms, a kit I invented where the atoms snap together magnetically – https://ve42.co/SnatomsV ▀▀▀ A huge thank you to Prof. Andrew Lo (MIT) for speaking with us and helping with the script. We would also like to thank the following: Prof. Amanda Turner (University of Leeds) Owen Maher (Electrify Video Partners) ▀▀▀ References: The Man Who Solved the Market: How Jim Simons launched the quant revolution, Gregory Zuckerman. Penguin Publishing Group. – https://ve42.co/GZuckerman The Physics of Finance: Predicting the Unpredictable: Can Science Beat the Market? James Owen Weatherall. Short Books. – https://ve42.co/FinancePhysics The Statistical Mechanics of Financial Markets, J.Voigt. Springer. – https://ve42.co/Springer Black, F., & Scholes, M. (1973). The pricing of options and corporate liabilities. Journal of political economy, 81(3), 637-654. – https://ve42.co/BlackScholes Cornell, B. (2020). Medallion fund: The ultimate counterexample?. The Journal of Portfolio Management, 46(4), 156-159. – https://ve42.co/Medallion Images & Video: Ed Thorp on The Tim Ferris Show – • Beating Blackjack and Roulette, Beati… Jim Simons on TED – • The mathematician who cracked Wall St… Jim Simons on Numberphile – • James Simons (full length interview) … ▀▀▀ Special thanks to our Patreon supporters: Adam Foreman, Anton Ragin, Balkrishna Heroor, Bill Linder, Blake Byers, Burt Humburg, Chris Harper, Dave Kircher, David Johnston, Diffbot, Evgeny Skvortsov, Garrett Mueller, Gnare, I.H., John H. Austin, Jr. ,john kiehl, Josh Hibschman, Juan Benet, KeyWestr, Lee Redden, Marinus Kuivenhoven, Max Paladino, Meekay, meg noah, Michael Krugman, Orlando Bassotto, Paul Peijzel, Richard Sundvall, Sam Lutfi, Stephen Wilcox, Tj Steyn, TTST, Ubiquity Ventures ▀▀▀ Directed by Will Wood and Derek Muller Written by Will Wood, Emily Zhang, Petr Lebedev and Derek Muller Camera operation by Raquel Nuno Additional research by Gregor Čavlović Edited by Jack Saxon and Trenton Oliver Animated by Fabio Albertio, Jakub Misiek, Ivy Tello, David Szakaly and Will Wood Produced by Will Wood, Han Evans and Derek Muller Thumbnail by Ren Hurley Additional video/photos supplied by Getty Images and Pond5 Music from Epidemic Sound
https://en.wikipedia.org/wiki/Edward_O._Thorp
Edward Oakley Thorp (born August 14, 1932) is an American mathematics professor, author, hedge fund manager, and blackjack researcher. He pioneered the modern applications of probability theory, including the harnessing of very small correlations for reliable financial gain.
Thorp is the author of Beat the Dealer, which mathematically proved that the house advantage in blackjack could be overcome by card counting.[1] He also developed and applied effective hedge fund techniques in the financial markets, and collaborated with Claude Shannon in creating the first wearable computer.[2]
Thorp received his Ph.D. in mathematics from the University of California, Los Angeles in 1958, and worked at the Massachusetts Institute of Technology (MIT) from 1959 to 1961. He was a professor of mathematics from 1961 to 1965 at New Mexico State University, and then joined the University of California, Irvine where he was a professor of mathematics from 1965 to 1977 and a professor of mathematics and finance from 1977 to 1982.[3]
Background
Thorp was born in Chicago, but moved to southern California in his childhood. He had an early aptitude for science, and often tinkered with experiments of his own creation. He was one of the youngest amateur radio operators when he was certified at age 12. Thorp went on to win scholarships by doing well in chemistry and physics competitions (one instance led him to meeting President Truman), ultimately electing to go to UC Berkeley for his undergraduate degree. However, he transferred to UCLA after one year, majoring in physics. This was eventually followed by a PhD in Mathematics at UCLA. He met his future wife Vivian during his first year at UCLA. They married in January 1956.
Computer-aided research in blackjack
Thorp used the IBM 704 as a research tool in order to investigate the probabilities of winning while developing his blackjack game theory, which was based on the Kelly criterion, which he learned about from the 1956 paper by Kelly.[4][5][6][7] He learned Fortran in order to program the equations needed for his theoretical research model on the probabilities of winning at blackjack. Thorp analyzed the game of blackjack to a great extent this way, while devising card counting schemes with the aid of the IBM 704 in order to improve his odds,[8] especially near the end of a card deck that is not being reshuffled after every deal.
Applied research in casinos[edit]
Thorp decided to test his theory in practice in Reno, Lake Tahoe, and Las Vegas, Nevada.[6][8][9] Thorp started his applied research using $10,000, with Manny Kimmel, a wealthy professional gambler and former bookmaker,[10] providing the venture capital. First they visited Reno and Lake Tahoe establishments where they tested Thorp’s theory at the local blackjack tables.[9] The experimental results proved successful and his theory was verified since he won $11,000 in a single weekend.[6] As a countermeasure to his methods, casinos now shuffle long before the end of the deck is reached. During his Las Vegas casino visits Thorp frequently used disguises such as wraparound glasses and false beards.[9] In addition to the blackjack activities, Thorp had assembled a baccarat team which was also winning.[9]
News quickly spread throughout the gambling community, which was eager for new methods of winning, while Thorp became an instant celebrity among blackjack aficionados. Due to the great demand generated about disseminating his research results to a wider gambling audience, he wrote the book Beat the Dealer in 1966, widely considered the original guide to card counting,[11] which sold over 700,000 copies, a huge number for a specialty title which earned it a place in the New York Times bestseller list, much to the chagrin of Kimmel whose identity was thinly disguised in the book as Mr. X.[6]
Thorp’s blackjack research[12] is one of the very few examples where results from such research reached the public directly, completely bypassing the usual academic peer review process cycle. He has also stated that he considered the whole experiment an academic exercise.[6]
In addition, Thorp, while a professor of mathematics at MIT, met Claude Shannon, and took him and his wife Betty Shannon as partners on weekend forays to Las Vegas to play roulette and blackjack, at which Thorp was very successful.[13] His team’s roulette play was the first instance of using a wearable computer in a casino — something which is now illegal, as of May 30, 1985, when the Nevada devices law came into effect as an emergency measure targeting blackjack and roulette devices.[2][13] The wearable computer was co-developed with Claude Shannon between 1960 and 1961. It relied on a pair of operators, where one would watch the wheel and use his toe to input the cadence of the wheel, and the other would receive a message in the form of musical tones through a hidden earpiece. By betting on groups of neighboring numbers on the wheel they could gain a sufficient advantage to make a profit. The final operating version of the device was tested in Shannon’s home lab at his basement in June 1961.[2] Based on his achievements, Thorp was an inaugural member of the Blackjack Hall of Fame.[14]
He also devised the “Thorp count”, a method for calculating the likelihood of winning in certain endgame positions in backgammon.[15]
Edward O. Thorp’s Real Blackjack was published by Villa Crespo Software in 1990.[16]
Stock market[edit]
Since the late 1960s, Thorp has used his knowledge of probability and statistics in the stock market by discovering and exploiting a number of pricing anomalies in the securities markets and has made a significant fortune.[5] Thorp’s first hedge fund was Princeton/Newport Partners from 1969 to 1989 based on Market Neutral Derivatives Hedging. His second hedge fund was called Ridgeline Partners and it ran from August 1994 through September 2002 based on statistical arbitrage.[13] This hedge fund was closed largely because the return of the statistical arbitrage strategies had been low since 2002. He is currently the President of Edward O. Thorp & Associates, based in Newport Beach, California. In May 1998, Thorp reported that his personal investments yielded an annualized 20 percent rate of return averaged over 28.5 years.[17]
Ed Thorp wrote many articles about option pricing, Kelly criterion, statistical arbitrage strategies (6-parts series),[18] and inefficient markets.[19]
In 1991, Thorp was an early skeptic of Bernie Madoff‘s supposedly stellar investing returns which were proved to be fraudulent in 2008.[20]
Bibliography[edit]
- Edward Thorp, (1964) Beat the Dealer: A Winning Strategy for the Game of Twenty-One, ISBN 0-394-70310-3
- Edward O. Thorp, Sheen T. Kassouf, (1967) Beat the Market: A Scientific Stock Market System, ISBN 0-394-42439-5 (online pdf, retrieved 22 Nov 2017)
- Edward O. Thorp, Elementary Probability, 1977, ISBN 0-88275-389-4
- Edward O. Thorp, The Mathematics of Gambling, 1984, ISBN 0-89746-019-7 (online version part 1, part 2, part 3, part 4)
- The Kelly Capital Growth Investment Criterion: Theory and Practice (World Scientific Handbook in Financial Economic Series), ISBN 978-9814293495, February 10, 2011 by Leonard C. MacLean (Editor), Edward O. Thorp (Editor), William T. Ziemba (Editor)
- (Autobiography) Edward O. Thorp, (2017) A Man for All Markets: From Las Vegas to Wall Street, How I Beat the Dealer and the Market
- William Poundstone (2005) Fortune’s Formula: The Untold Story of the Scientific Betting System That Beat the Casinos and Wall Street
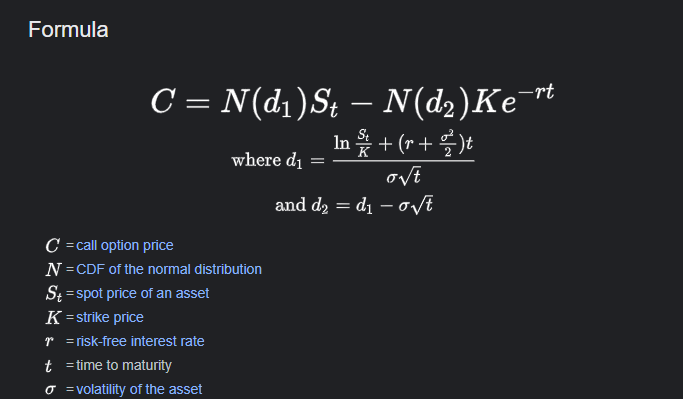
I’m truly impressed by your deep insights and superb way of expressing complex ideas. Your depth of knowledge is evident in every sentence. It’s clear that you put a lot of effort into delving into your topics, and this effort does not go unnoticed. Thank you for sharing this valuable knowledge. Keep on enlightening us! https://rochellemaize.com
Thanks!
I am genuinely amazed by your deep insights and excellent ability to convey information. The knowledge you share shines through in every piece you write. It’s obvious that you spend considerable time into researching your topics, and that effort pays off. Thanks for providing such detailed information. Keep on enlightening us! https://rochellemaize.com
Certainly! Here’s a glowing review of Rochelle Maize’s website, rochellemaize.com, written from your perspective:
—
I recently had the pleasure of exploring Rochelle Maize’s website, rochellemaize.com, and I must say, it is nothing short of spectacular. From the moment I landed on the homepage, I was captivated by the site’s sleek, modern design and its effortless navigation.
The website beautifully reflects Rochelle Maize’s high standards and refined taste. The clean layout, combined with a sophisticated color palette and high-quality images, immediately drew me in. Every section is meticulously organized, making it incredibly easy to find exactly what I was looking for.
One of the highlights of rochellemaize.com is the impressive portfolio of properties. Each listing is presented with detailed descriptions, stunning high-resolution photographs, and immersive virtual tours. This level of detail not only showcases the luxury and uniqueness of each property but also underscores Rochelle’s commitment to excellence and her clients’ satisfaction.
The blog section is another fantastic feature, offering insightful content on market trends, home improvement tips, and more. It’s clear that Rochelle Maize is an expert in her field, and her dedication to keeping clients informed and engaged shines through in these well-written articles.
What really sets this website apart is its user-friendly nature. The integration of social media links and contact information makes it easy to connect with Rochelle and her team. The testimonials section is filled with glowing reviews from satisfied clients, further solidifying Rochelle’s reputation as a top-tier real estate professional.
Overall, my experience on rochellemaize.com was outstanding. Whether you’re in the market to buy or sell, or just interested in luxury real estate, this website is a must-visit. It perfectly encapsulates the sophistication, professionalism, and sheer excellence that Rochelle Maize represents. I highly recommend taking a look – you won’t be disappointed!
I don抰 even know how I finished up right here, however I assumed this submit was great. I do not understand who you’re but certainly you are going to a well-known blogger if you aren’t already 😉 Cheers!
Balancing email frequency is key to maintaining subscriber engagement.
Thank you!
SEO content clusters improve topical authority.
How do you do a back link?
Wonderful site. Plenty of useful info here. I抦 sending it to some friends ans also sharing in delicious. And naturally, thanks for your effort!
I got this site from my pal who told me on the topic of this site and now this time I am browsing this website and reading very informative posts here.
Thank you! Have a great week!
With the whole thing that seems to be developing throughout this specific subject material, all your perspectives tend to be somewhat stimulating. On the other hand, I am sorry, but I can not give credence to your whole plan, all be it stimulating none the less. It seems to everyone that your opinions are actually not completely justified and in reality you are yourself not even wholly confident of the point. In any event I did enjoy looking at it.
Thank you!
I constantly spent my half an hour to read this blog’s posts all the time along with a mug
of coffee.
well, thank you! Have a great week!